If you consider a Wiener process Wₜ, and multiply it by its integral ∫ from 0 to t Wₛ ds, you get a product of two stochastic processes: Wₜ ⋅ ∫ from 0 to t Wₛ ds.
The product Wₜ ⋅ ∫ from 0 to t Wₛ ds is a nonlinear function of the Wiener process. In stochastic calculus, dealing with nonlinear functions of stochastic processes generally requires tools like Itô's lemma, which allows for the differentiation and integration of such functions.
The product Wₜ ⋅ ∫ from 0 to t Wₛ ds is a stochastic process itself and inherits the randomness from the Wiener process. Its behavior is more complex than either Wₜ or ∫ from 0 to t Wₛ ds alone, due to the interaction between the instantaneous value of Wₜ and its accumulated past values.
This product also relates to the concept of quadratic covariation, which is a measure of how two stochastic processes co-vary in a quadratic sense. In the case of Wₜ and its integral, analyzing their quadratic covariation would be part of understanding their joint behavior.
In the context of the product of a Wiener process Wₜ and its integral, the quadratic covariation helps in understanding the interaction between the Wiener process at a point in time and its accumulated effect over time, in a more nuanced way than simple linear covariation. This quadratic aspect becomes particularly relevant in complex financial instruments like Asian options, where such intricate relationships play a key role in pricing and risk assessment.
The Wiener process, also known as Brownian motion, is often described as "memoryless" in that its increments are independent. This means the future behavior of the process depends only on its current state and not on its history. Formally, for any s < t, the increment Wₜ - Wₛ is independent of the process's history up to time s.
The integral ∫ from 0 to t Wₛ ds, however, is a cumulative measure. It sums up the values of the Wiener process over time, inherently incorporating the process's history up to time t. This integral is not memoryless because it aggregates past information.
Multiplying Wₜ with its integral combines a memoryless process at a specific point in time with a cumulative process that inherently contains historical information. This product captures an interaction between the current state, which is memoryless in isolation, and the aggregate effect of the process's history.
The product Wₜ ⋅ ∫ from 0 to t Wₛ ds can be seen as a reflection of how the current state, memoryless at the moment, is influenced when considered in conjunction with the accumulated path it has taken, which is not memoryless.
This nuanced interaction is particularly relevant in the field of financial mathematics, where such constructs can be used to price exotic options, such as Asian options whose payoff is path-dependent.
#StochasticProcesses #ItôsLemma #StochasticCalculus #QuadraticCovariation #BrownianMotion
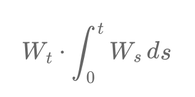
Écrire commentaire