Why Cov(W_s, W_t) = min{s, t} in Simples Terms

Key Takeaways:

• A Wiener process is like a random walk; Sam and Tina’s steps are random, similar to coin flips.
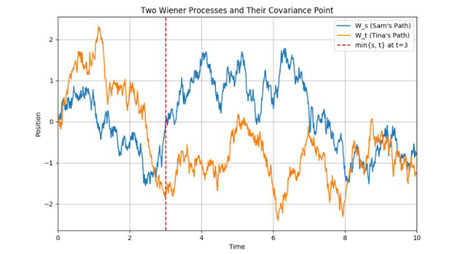
• Cov(W_s, W_t) = min{s, t} represents their joint movement over time.
• Their covariance only covers the shared time walking; any extra steps beyond that don’t count.
• The shared duration of their walk, not individual steps, determines their joint variability.
• The variance of their paths is tied to the time they walk together, emphasizing their intertwined journey.

Standard Wiener processes sound complicated, but imagine it as a random walk in a park. Picture two friends, Sam and Tina, taking such a walk.

Both Sam and Tina start at the same location in the park and begin their walk simultaneously. Their next step is entirely random, much like flipping a coin: a head might mean taking a step forward while a tail could signify stepping back.

The mathematical relationship that captures the joint randomness or "connectedness" of their walks is denoted by the equation: Cov(W_s, W_t) = min{s, t}.

Covariance is a concept that evaluates the joint variability of two processes. Essentially, it provides insights into how two paths (here, Sam's and Tina's) move relative to one another.

W_s reflects where Sam is at time (s), and W_t represents Tina's position at time (t).

Now, the interesting part is how this equation behaves:

1. If Sam's random walk lasts 5 minutes (s=5) but Tina stops at 3 minutes (t=3), then the overlapping time they've actually spent walking together is only those first 3 minutes. 

2. Any deviation or random step Sam takes after these 3 minutes doesn't affect or is unrelated to Tina's walk since she has already stopped.

The formula, Cov(W_s, W_t) = min{s, t}captures this essence. It's not about how long each one walks but about the time they spend walking together. 

In mathematical jargon, the paths of Sam and Tina are said to "co-vary" or exhibit joint fluctuations only during the period they both are walking. The covariance emphasizes the shared journey duration, which is always the shorter of the two time periods.

Interestingly, despite the independent randomness of their steps, the only factor influencing their joint movement is time, not the steps themselves. This is because the variance of each Wiener process, or the measure of their individual unpredictability, is directly proportional to time. 

Thus, the formula highlights that Sam and Tina's combined randomness is confined to the time they share in their journey, representing how their paths intertwine during that period.

The relationship is indeed about the shared duration of the two paths and their joint variability within that timeframe, rather than the specifics of their individual movements akin to the covariance of two standard Wiener processes.